Thermodynamic potentials are used to measure the energy of a system in terms of different variables because often we can only measure certain properties of the system. For example we might know the pressure and temperature of system but not the volume or the entropy. Thermodynamic potentials allow us to measure more state variables of the system.
The thermodynamics potentials are the internal energy, enthalpy, Gibbs Free Energy and the Helmholtz Free Energy.
These different potentials can be remembered by writing them in the form of a grid.
| +P V | ||
| U | H | |
| –T S | F | G |
From the first law of thermodynamics, the internal energy, is given by
dU = dQ + dW
dU = T dS + P dV
From H = U + P V, then dH = dU + dP V + PdV (using the chain rule).
The first column of the first row contains just the internal energy U, to obtain the enthalpy, we must add P V. In the second row, the Helmholtz Free F energy is the internal energy, U minus T S, finally in the second column of the second row, the Gibbs Free Energy G we must add P V and take away T S.
We wish to have a number of expressions for the energy of a system in terms of different variables, for we shall sometimes know the pressure and temperature of a system, but not the volume or entropy; or we might know the volume and temperature but not the pressure and entropy. In most cases, we shall know only a few relevant quantities and will wish to find out many more. This leads to the following definitions two or more thermodynamic quantities:
U
H = U + P V
F = U – T S
G = U + P V – T S
Using Thermodynamic Potentials
From the first and second laws of thermodynamics, dU and dQ are not obviously measurable. Therefore we need thermodynamic manipulation to get useful properties from what is measurable, i.e., P, V and T. Thermodynamics quantities are more commonly defined in terms of partial differentials. For example:
INTERNAL ENERGY
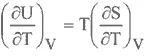
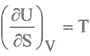
ENTHALPY
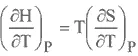
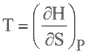
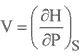
HELMHOLTZ FREE ENERGY
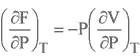
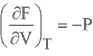
GIBBS FREE ENERGY
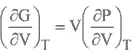
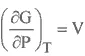


Comments are closed.